Art and Math

Designing for Considering Boundaries around Infinity

Paula Beardell Krieg
Thinking about squares and infinity

This was bound to happen, that I would put up a post on my book and paper arts blog that appears to just be about math.

Initial impressions sometimes need refinement.

Anyone who follows me has probably noticed my attention to math ideas emerging as a theme. I’ve been paying attention to math and it is shaping how my creative work is evolving.

What I am here to say right now is that I think that math needs more designers and paper engineers.

In calculus there is this thing called Integration-by-Parts. It requires either a fluency with many rules or access to tables that contain these rules. The two problems with this is that it is not typical for the student to be fluent with the rules and the tables are not at all friendly looking and are embedded in humongous textbooks

When I was doing integration by parts problems it occurred to me to make a foldable that organized the information I needed to do these problems into a handy reference page.

I got lots of input and help from folks in the math community. I now have a greater appreciation for people who write whole textbooks, as just this one foldable was a big deal to do.

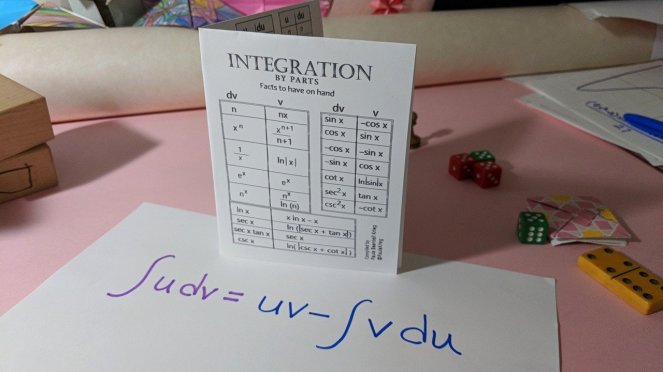
four page booklet
four page booklet

Here are the PDFs for

Addendum: If you have ever fantasied about have an Integration by Parts beach towel, know that some dreams can come true: https://society6.com/product/integration-by-parts_beach-towel?sku=s6-11177199p53a69v456

I’m going to be making a series of videos integration-by-parts.  As they are done, I will be editing them into this post so that I don’t flood my book arts followers with math videos. Still, I hope some of my non-math friends will take a look at this up to the 6:05 minute mark and tell me if it makes sense to them at all.  I am really enjoying being an artist who thinks about math instruction as a design issue.

Much of my thinking about math, as in my thinking about book arts instruction, centers around the weak links, meaning I search for places where misunderstanding sabotages learning.

This next video tries to address the disorientation of no longer solving for x, after solving for x for so many years.

Here’s the third video. At this point there’s not much here of general interest as it’s getting more specific to this specific method.

Finally, some worked examples. So far I’m showing two problems, but hope to add two more in the near future. Then these will be done!

The one below has a bit of bonus material about Desmos.com near the beginning.

Published by Paula Beardell Krieg

Visual artist Paula Beardell Krieg has been teaching bookmaking to students in schools, to teachers and to other artists since the mid 1980's.

4 thoughts on “Designing for Considering Boundaries around Infinity”

  1. I suspect that more artists think about math than many people realise. It can be fairly obvious in things like origami, but I had a friend who was a mathematician-turned-basketmaker, who sometimes made baskets that were physical representations of mathematical formulae. (Not that everyone could see that!)

    Like

    Reply

    1. I agree with you that probably many artists think about math. Many times I’ve read bios about women artist who started college with the intention of going into math. Something I realized last week, while I was drawing daffodils…a drawing is created by lines that exist in relationships to each other, generally to describe an object. Similarly, equations and graphs are created to describe observations of a different kind by using numbers that describe relationships between numbers. I probably haven’t said that well, but the point is that both drawings and equations are in the business of description. Math and art have more in common than is immediately evident..

      Liked by 1 person

      Reply

Leave a comment